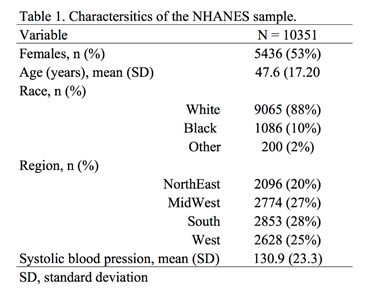
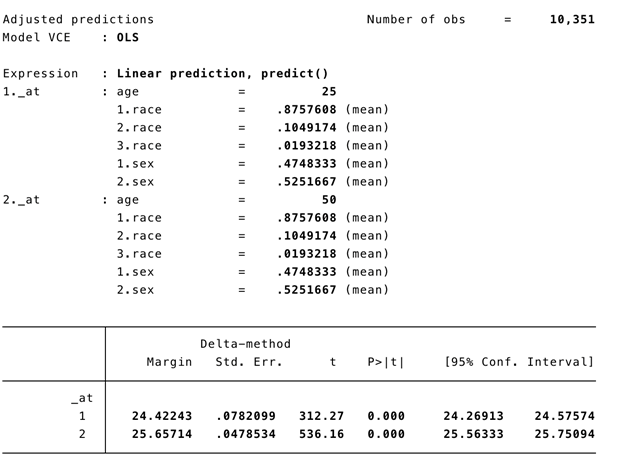
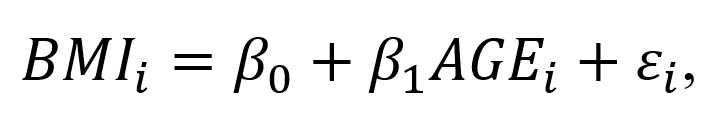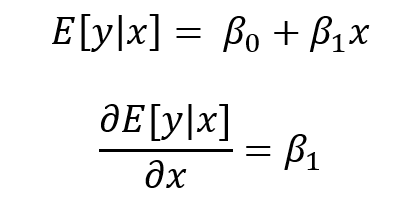BACKGROUND
As the COVID-19 pandemic, which began in December 2019, continues into its second year, public health measures have been put into place to mitigate its spread. At the time of writing this article, there have been over 4.5 million deaths and over 216 million cases due to COVID-19.[1] Surveillance of COVID-19 remains an important public health measure of understanding the spread and impact. Daily reports such as the John Hopkins COVID-19 dashboard provide end users with visual and statistical information about the surges in cases and deaths associated with COVID-19. However, one measure that is of great interest is the reproduction number or R0.
Reproduction number (R0) and effective reproduction number (Rt)
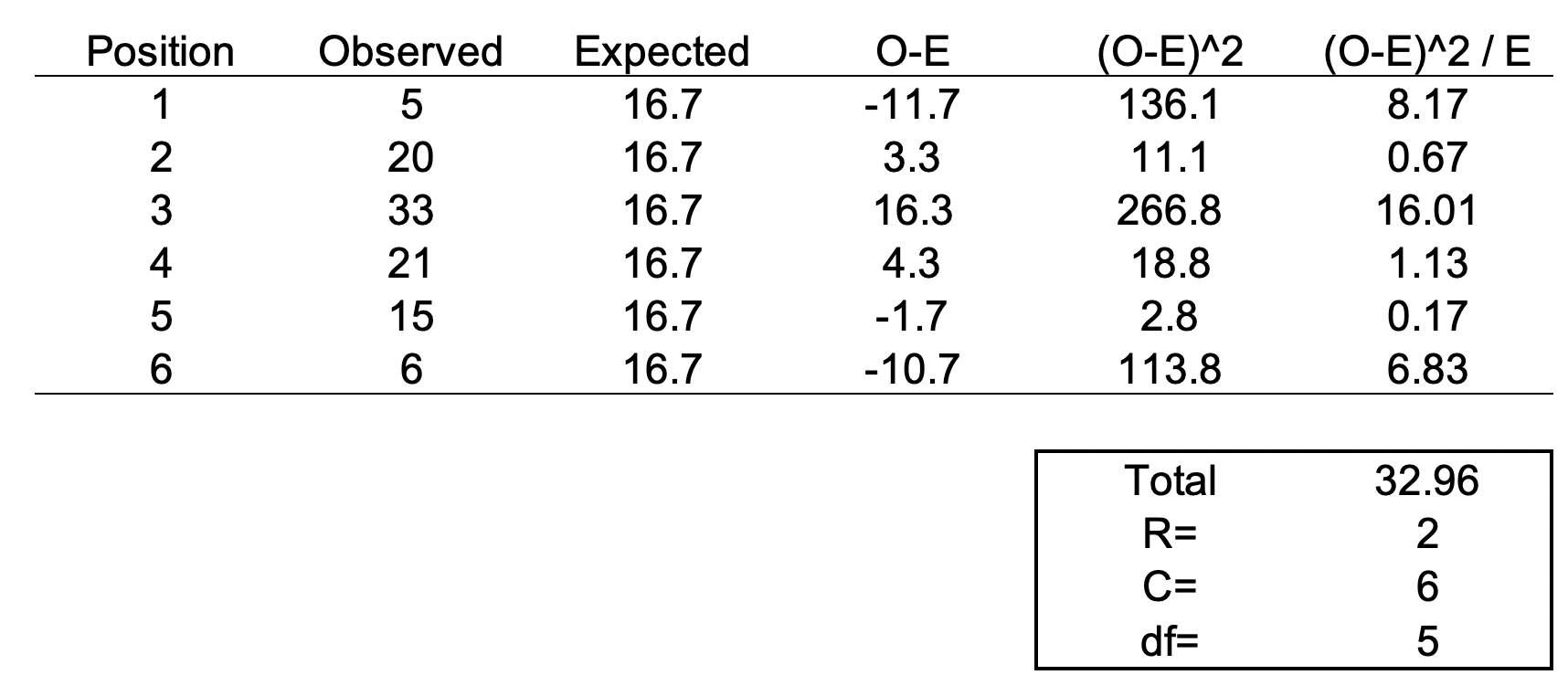
The reproduction number is the number of new cases that is directly caused by exposure to a single case.[2,3] Figure 1 provides a visual explanation of the basic reproduction number. However, the underlying assumption with R0 is that everyone in the population is susceptible to infection. With the introduction of vaccines, the R0 isn’t a good measure of the reproductive capabilities of COVID-19. Instead, the effective reproduction number (Rt) is used to provide a more realistic reproduction number based on the population being infected, recovered, or vaccinated. The Rt changes over time as the population susceptible to infection changes.
Figure 1. Basic reproduction number.
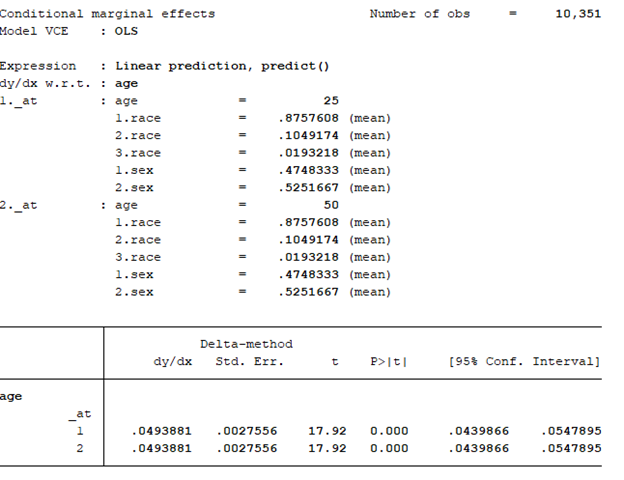
I wanted to create a figure that would highlight the changes associated with the Rt for each state in the United States. To do this, I downloaded the Rt data from the by Xihong Lin's Group in the Department of Biostatistics at the Harvard T.H. Chan School of Public Health. They have an amazing COVID-19 tracker dashboard that captures the changing patterns of Rt for each state. Then I created a Cleveland plot to show where the Rt was near the beginning of the pandemic and where it is currently (August 2021). (Note: I wrote a tutorial on creating Cleveland plots that you can review here.) Here is the final figure (because of the length of the figure, I cropped it to show the first 30 states or territories):
Figure 2. Effective reproduction number (Rt) for U.S. states and territories, April 17, 2020 (past) to August 14, 2021 (recent).
The blue dots denote the most recent effective reproduction number (14 August 2021) and the past dots denote the earliest effective reproduction number (17 April 2020).
It seems that some states have gotten worse in terms of increase effective reproduction number since the beginning of the pandemic. This could be due to lack of good data in the early phases of the pandemic. However, what is of concern is the high effective reproduction numbers in some states (Rt > 2), which indicates that the pandemic is still spreading at an alarming rate.
There were some missing data which are identified by a single dot (blue or red) or an empty field in the recent or past effective reproduction number. Rather than fill these in, I left them empty. There may be data in between the two time periods that I could have used, but I left those out.
One thing to mention is that this Cleveland plot only tells us one dimension of the effective reproduction number story (the difference between the most recent Rt and the earliest Rt). It doesn’t tell us much about how the effective reproduction number changes across time. For that, I direct your attention to the Lin’s Laboratory Group at Harvard, they have a great figure that shows the fluctuation of the effective reproduction number for the U.S. and its states/territories (see example):
Source: Lin’s Laboratory Group at Harvard (link). [last accessed on 30 August 2021].
CONCLUSIONS
The effective reproduction number provides us with some interesting patterns in spread of COVID-19 by states/territories. It seems to have worsened over time, but this could be due to poor data early in the pandemic. There are some issues with the us of effective reproduction number for policy decisions. Reporting delays can impact the estimates for the effective reproduction number. A technique called “nowcasting” is used to estimate the reproduction number.[3] But when I explored some of the work in this area, there appears to be a variety of methods for performing this technique. Despite this limitation, the effective reproduction number may be useful to evaluate public health policy decisions to reduce the spread of the COVID-19 pandemic.[4,5]
DATA SOURCE
I provided the link to the COVID-19 Spread Tracker from the Lin Lab at Harvard. You can also download a curated version of the data for this article from my Dropbox folder. The data are current as of 17 August 2021. If you’re interested in recreating this Cleveland plot, I recommend downloading the most recent data to see how much the effective reproduction number has changed.
REFERENCES
Worldometeres.info. COVID Live Update: 217,770,381 Cases and 4,521,936 Deaths from the Coronavirus - Worldometer. Accessed August 30, 2021. https://www.worldometers.info/coronavirus/
Lim J-S, Cho S-I, Ryu S, Pak S-I. Interpretation of the Basic and Effective Reproduction Number. J Prev Med Pub Health. 2020;53(6):405-408. doi:10.3961/jpmph.20.288
Adam D. A guide to R — the pandemic’s misunderstood metric. Nature. 2020;583(7816):346-348. doi:10.1038/d41586-020-02009-w
Inglesby TV. Public Health Measures and the Reproduction Number of SARS-CoV-2. JAMA. 2020;323(21):2186-2187. doi:10.1001/jama.2020.7878
Pan A, Liu L, Wang C, et al. Association of Public Health Interventions With the Epidemiology of the COVID-19 Outbreak in Wuhan, China. JAMA. 2020;323(19):1915-1923. doi:10.1001/jama.2020.6130